Embark on an enlightening journey with the Practice and Homework Lesson 11.3 Answer Key, your definitive guide to comprehending geometric transformations. This key unveils the fundamental concepts, applications, and real-world significance of transformations, empowering you to master this captivating mathematical domain.
Delve into the intricacies of translations, rotations, and reflections, gaining a profound understanding of their properties and effects on geometric figures. Explore the concept of inverse transformations, unlocking the ability to reverse the impact of transformations and restore original configurations.
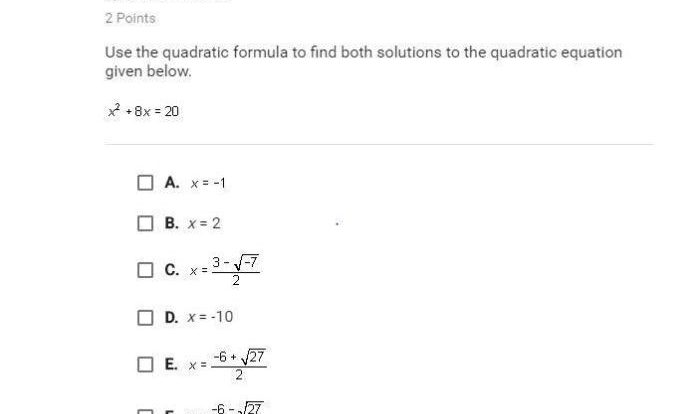
Practice and Homework Lesson 11.3: Understanding Transformations
Lesson 11.3 introduces the fundamental concepts of transformations, providing a solid foundation for understanding how geometric figures can be manipulated and transformed. Transformations are operations that move, rotate, or reflect figures, and they play a crucial role in various fields, including geometry, engineering, and computer graphics.
Types of Transformations
- Translation:Moves a figure from one point to another without changing its size or shape.
- Rotation:Turns a figure around a fixed point by a specified angle.
- Reflection:Flips a figure over a line, creating a mirror image.
Properties of Transformations
Transformations possess certain properties that govern their behavior:
- Preserve Distance:Translations and rotations preserve the distance between points in a figure.
- Preserve Orientation:Rotations and reflections preserve the orientation of a figure, meaning they do not change its “handedness” (left-right or up-down).
Applying Transformations to Geometric Figures: Practice And Homework Lesson 11.3 Answer Key
Transformations can be applied to geometric figures of various shapes and sizes, including points, lines, and polygons.
Effects of Transformations on Different Figures
| Transformation | Point | Line | Triangle |
|---|---|---|---|
| Translation | Moves the point | Moves the line | Moves the triangle |
| Rotation | Rotates the point | Rotates the line | Rotates the triangle |
| Reflection | Flips the point | Flips the line | Flips the triangle |
Inverse Transformations
Inverse transformations are operations that undo the effects of transformations. For example, the inverse of a translation is another translation in the opposite direction.
Composing Transformations
Transformations can be composed, meaning they can be applied in succession to create more complex transformations.
Examples of Composed Transformations, Practice and homework lesson 11.3 answer key
- Translation followed by a rotation:Moves a figure and then rotates it around a point.
- Rotation followed by a reflection:Rotates a figure and then flips it over a line.
Properties of Composed Transformations
Composed transformations inherit certain properties from the individual transformations they are composed of:
- Order of Transformations:The order in which transformations are composed affects the final result.
- Identity Transformation:The identity transformation is a transformation that does not change a figure.
Transformations in the Real World
Transformations find practical applications in various fields:
Examples of Transformations in Real-World Applications
- Architecture:Designing buildings and structures that involve rotations, reflections, and translations.
- Engineering:Analyzing the movement of objects in space and designing mechanical systems that undergo transformations.
- Computer Graphics:Creating 3D models, animations, and other visual effects that involve transformations.
Question Bank
What is the concept of transformations in geometry?
Transformations are operations that map geometric figures onto new positions or orientations while preserving their essential properties, such as size and shape.
What are the different types of transformations?
Common types of transformations include translations (shifting), rotations (turning), reflections (flipping), and dilations (scaling).
How are transformations applied to geometric figures?
Transformations can be applied to points, lines, and shapes, resulting in new figures with altered positions, orientations, or sizes.
What is the significance of inverse transformations?
Inverse transformations undo the effects of original transformations, restoring figures to their original configurations.